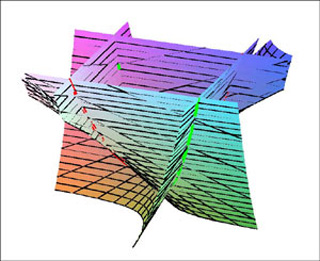
The discriminant of the polynomial x4 + 4ax3 + 6bx2 + 4cx + 1. The convex set inside the "bowl" corresponds to the region of nonnegativity. There is an additional one-dimensional component inside the set. (Image courtesy of Prof. Pablo Parrilo.)
Instructor(s)
Prof. Pablo Parrilo
MIT Course Number
6.972
As Taught In
Spring 2006
Level
Graduate
Course Description
Course Features
Course Description
This research-oriented course will focus on algebraic and computational techniques for optimization problems involving polynomial equations and inequalities with particular emphasis on the connections with semidefinite optimization. The course will develop in a parallel fashion several algebraic and numerical approaches to polynomial systems, with a view towards methods that simultaneously incorporate both elements. We will study both the complex and real cases, developing techniques of general applicability, and stressing convexity-based ideas, complexity results, and efficient implementations. Although we will use examples from several engineering areas, particular emphasis will be given to those arising from systems and control applications.


