Flash and JavaScript are required for this feature.
Download the video from iTunes U or the Internet Archive.
Topics covered: Le Chatelier's principle and applications to blood-oxygen levels
Instructor: Catherine Drennan, Elizabeth Vogel Taylor

Lecture 20: Le Chatelier's ...
Related Resources
Lecture Notes (PDF)
The following content is provided under a Creative Commons license. Your support will help MIT OpenCourseWare continue to offer high quality educational resources for free. To make a donation or view additional materials from hundreds of MIT courses, visit MIT OpenCourseWare at ocw.mit.edu.
PROFESSOR: OK, it's time to get started. Pay attention to the clicker questions. All right, I'll give you 10 more seconds. Not bad, we seem to be in the 70's. All right. So if you didn't have time to click in, let's consider what's going on here.
What's true about the relationship of q and k here? So is q less than or greater than k? Less than. And so you have to think about what that means in terms of where we are now in the reaction and where the reaction is at equilibrium, and I like to think about it in terms of products -- are there more products now, or are there more products at equilibrium. So if q is less than k, then there are more products at equilibrium, and so the reaction will shift toward products. So it's going to move in the direction to reach equilibrium, since k is a bigger number than q, and both of these terms are products over reactants, there'd mean there's more product at equilibrium then there are now, and so the reaction is going to shift toward products.
All right, so we're at 73%. Let's see if by the end of today, we can get up to 90 on these kinds of questions.
All right, so we're going to continue to consider external effects on k in today's class. I also wanted to mention that on each of the handouts for the lectures, I have the corresponding reading material listed on the handout, and I've listed it as section numbers in the chapters rather than page numbers, so that's a little bit of a difference. And the reason why I've done that is that we've had many different versions of the book in this class, and they don't seem to change the section titles or the section numbers, but they do change the page numbers associated with them. So if I give you section numbers then you should be able to use of whichever version of the book is available to you. So that's how I have it listed now, and the reading assignment is also listed on each problem-set as well. So when you're going over the handouts later studying for the exam, you can see where in the book this particular lecture, the material is, so you can go and do that reading.
All right, so we talked at the end of the class last time about the Le Chatelier's principle, and this is a principle that you can use to predict the direction of change of a reaction -- whether it'll shift to the right or shift to the left. And simply stated, that systems tend to respond in such a way to minimize the stress. So if something is added to the reaction, the reaction will shift in a way to minimize that stress caused by the thing that's added to the system.
So, we talked about adding reagents and removing products last time, and now we're going to go on and talk about what happens when you change the volume. So if you have a system at equilibrium and you change the volume of it, and here in particular we're talking about a gaseous system, what's going to happen?
So what do we know about volumes when it comes to gases? We say that a decrease in volume of the gaseous system causes an increase in total pressure. What equation leaps to your mind when I say those words? Exactly, p v equals n r t. Yes. So there's a relationship between pressure and volume -- n is the number of moles, r is our gas constant, and t is our temperature. This is one of the things that usually sticks from high school, and if you haven't seen it before, it's pretty easy to get up to speed with this equation.
So Le Chatelier's principle then predicts that the system will respond if possible in a way to reduce the total pressure. So if you decrease the volume and you cause an increase in total pressure, Le Chatelier says wait a minute, the system wants to respond to minimize that stress, so that it's going to respond in such a way to reduce that total pressure. So let's look at some examples of this.
So we have an example of a reaction where we have two moles of p 2 gas going to one mole going to p 4 gas. And I have a little cartoon here to show this. So we have our p 4 gas up here, and our p 2 gas. All right, so what's going to happen if we're going to change the volume of this system? So if we're going to decrease the volume of the system, then the reaction should shift toward products. So let's think about that. So here in the middle we have our sort of system, and then on one side what happens when you decrease the volume and on the other side we're expanding the volume. So first, let's consider our system when we're going to compress that volume, decrease that volume. So what would be true is that the system's going to respond in such a way to minimize that stress that has been put forward by decreasing the volume, and you're going to try to compensate for that, and in this case you can compensate by a shift toward products. Why is that? Well, it all has to do with the stoichiometry of this equation up here. So for every two moles of reactants, you get one mole of products. So if you shift from the two to the one, that will cause a decrease in pressure, and so that will help compensate for the increase in pressure caused by the switch in volume. So you're going to respond in such a way to compensate for that stress. So a shift to the reaction to the right decreases the total pressure.
So now let's think more about why this is true. Let's think about it from a math perspective and also just this qualitative perspective. So let's consider it in terms of q and k. So suppose our volume is decreased by a factor of 2, let's just make it easy, and we have constant temperature here. So this change will increase the partial pressure of both gases, the reacting gas and the product gas, and it's going to increase them both by the same amount, by 2 initially.
So let's look at our equation for q, the reaction quotient. We have partial pressure of products over the partial pressures of the reactants, a raise to the coefficient to the equation. So initially you're going to increase the pressure, partial pressure of both gases by 2 -- then your product by 2, the reactant by 2 -- but here it's 2 squared. So overall, q becomes 1/2. So q decreases by a factor of 2, and so q becomes less then k. And so, in the clicker question, we talked about what happens when q becomes less than k, and what happens there is you're going to shift toward products until q equals k again.
So you can think about this in terms of q and k, and you can also just think about it how many moles of gas are on one side, how many mold of gas on the other side, and how are you going to correspond, how are you going to decrease or minimize the stress on the system.
All right, so let's think about what happens if we increase the volume, so here is our system and now we're going to expand it. If we're going to increase the volume, what's going to happen to the total pressure? I'm hearing it, but I want everyone to answer. Thank you. All right, so it will decrease the pressure. And what that's going to do is shift toward reactants, because we want to compensate for that decrease in pressure, and to do that, we can increase the pressure by switching or shifting the reaction toward the reactants. So again, we're going from one mole of product on one side to two of the reactants. So here, we see a shift toward the reactants. And so, this shift to the left is going to increase the total pressure to compensate for the decrease in pressure that was a result of this applied force to the system. And we could do this again in terms of q and k. Now we're going to get a little trickier. What happens if we add an inert gas to a system increasing the total pressure at constant temperature? So we're not adding one of the reactants or one of the products, we're adding an inert gas, and we're increasing the total pressure. What's going to happen? There's a couple of options, what do you think? How about nothing. Why would nothing happen? Well, q depends on the partial pressure of the reactant gas and the product gas. And in this particular example, the partial pressures are not changing. We are changing the total pressure of the system by adding an inert gas, but we're not changing the partial pressure.
So let's take this opportunity to review partial pressures, or if you haven't seen it before, I'm going to tell you everything you need to know about partial pressures. So here's a little review, or for the first time, description of partial pressure.
So what is the definition? The partial pressure is the pressure that each gas would exert if it were present alone in the container. So in this example, we have oxygen in a container, and we have one atmosphere of pressure. In the second one there's nitrogen in the container with one atomosphere of pressure. If you put this amount of oxygen and this amount of nitrogen together in a container, then you would have two atmospheres of total pressure, but you're still going to have one atmosphere of partial pressure for oxygen, and one atmosphere of partial pressure for nitrogen. So it's as if the gas is alone in the container. That's the definition of partial pressure. So let's look at some equations.
So the partial pressure of a gas, p to sub a, is equal to the number of moles of that gas, and we also have in this equation, r, our gas constant, t, our temperature, and v, our volume. The total pressure on the system, which is what this two atmospheres is, is equal to the partial pressure of gas a, which would be the partial pressure of the oxygen at one atmosphere, the partial pressure of the nitrogen at one atmosphere, that's all we have here. So the partial pressure of those two, of one plus one, we have two for a total. And that's equal to the total number of the moles.
So in these problems, we're going to be considering partial pressure, and the question you're going to be asking yourself in all of these different wordings of the question is is the partial pressure of the gas changing? That's a very important question to ask because that'll help you answer the questions correctly.
So let's go back to the original question, what happens if an inert gas is added to the container, increasing the total pressure at constant temperature? And the answer is nothing, because q is not affected, because q depends on the partial pressures, and the partial pressure isn't changing. We aren't changing the number of moles of the gas in question, we're just changing the total pressure of the system, we're adding an inert gas. And because partial pressures don't change, q doesn't change, and if q doesn't change, there's no response from the system.
So a lot of the questions in this unit, they're not actually very difficult, but the wording can be tricky. And so when you're doing these problems, you have to be thinking about what is changing and what isn't changing to be able to get these questions correct. And if you do that, then these are some nice questions for you to get right on the exams -- they're short, they're good questions to go after.
All right. Let's see how you're doing with this. What happens if an inert gas is added to the container, but the total pressure is now kept constant, temperature is also constant. So let's think about this. OK, let's take 10 more seconds. OK, we're going down into the 60's -- again, we're heading toward the 90's by the end, but this is actually new material, so that's fine.
All right, so let's think about it. So the reaction's going to shift toward reactants. Let's think about why, let's break it down. And what was important is that hint that for the pressure to be kept constant, the volume of the container must have increased. So let's take a look at that.
So if the total pressure was kept constant, if the total pressure didn't change when you added an inert gas, the volume must have changed. Let's look at this. Say oxygen is our gas of interest, and n 2 is an inert gas. If we added an inert gas to our system, the total pressure should go up. If it doesn't, then something else must have changed, and what must have changed is the volume. Otherwise the total pressure would not have stayed the same. So the volume of the container must have increased if the pressure is the same.
So then we asked the question, if you increase the volume, what happens to the reaction, how does it shift? If you increase the volume, if you expand the volume, then you're going to shift from, in this case where you have one mole to two moles toward reactants, you will, as the volume increases, then you're going to have a change in the partial pressure of the gas -- all of a sudden the gas has a lot more room, and its pressure is going to decrease, the partial pressure will decrease. It has a lot more space for itself -- it's like it's in there all by itself. Partial pressure, you can think of it as selfish gas molecules, they don't care what else is there. They're only thinking about how they're fairing in this environment. So then we want to shift in a way that compensates for that stress that increases the pressure, so we move from one molecule to two molecules.
So it's all about the partial pressure q and k. So again in these questions you have to dissect what's going on. What has changed? The questions are often phrased in such a way, something's kept constant, but if something's kept constant, you have to ask the question, to keep it constant, did something have to change for that to be true. So again, the wordings of these can be tricky.
Do you have a question?
STUDENT: [INAUDIBLE]
PROFESSOR: So, the question is is it necessary for it to try to increase pressure. This is just the predictive tool of Le Chatelier. Le Chatelier would predict that if the system has changed in such a way, such as volume increases so the partial pressure decreases, that the system will shift in a way to minimize that stress. And to minimize that stress, you would increase the partial pressure by doing the shift from one mole to two. So again, this is Le Chatelier's predictive, how you would predict the direction of the shift based on the simple idea that a system where a stress is applied, the system will respond in a way to minimize the stress.
And again, I talked about last time that this concept of minimizing stress is difficult for some MIT students, but you just sort of have to memorize that in this case we're predicting how a system will shift to minimize that stress.
OK. So if you need a review of partial pressure, go over this. This part, it looks a little challenging at first, and some of the questions you really have to sit down and dissect what's going on, and for most people, once they get there, that part, those are good questions, you look forward to them on the exam.
So now, we've talked about adding reagents, we've talked about removing reagents, removing products, adding products, shifts in volume, and now we're going to talk about changing the temperature. So here, Le Chatelier's principle, it's a little bit more fuzzy, but it still basically works. So if you talk about raising the temperature of a mixture at equilibrium, then Le Chatelier's principle would suggest that the system is going to respond in such a way to minimize that stress. So if you're adding heat, the system wants to respond in a way to absorb some of the heat, to counterbalance that change to the system. So let's take a look at this.
So let's think about raising the temperature of an exothermic reaction. So what's going to happen here? Raising the temperature of an exothermic reaction, favors the formation of reactants, would shift toward reactants. Well, why would this be true. Well, you can think about this very simplistically that in an exothermic reaction, you're going to be producing heat in the forward direction and absorbing heat in the reverse direction. So if it's an exothermic reaction, that means it's exothermic in the forward direction, which would mean it was endothermic in the reverse direction. So if you raise the temperature of an exothermic reaction, you're adding heat, the system wants to respond in such a way to absorb that heat, so it would shift toward the endothermic direction of the reaction, which is the reverse direction toward reactants. So add heat, system wants to shift to absorb some of that heat. So in this part you think about the direction of the reaction, whether it's exothermic or endothermic, and then think about compensating for that stress.
Endothermic reaction, again, they say it's an endothermic reaction, it means it's endothermic in the forward direction. So heat is being absorbed in the forward direction -- again this is just sort of a very simplistic way to think about it. So if you raise the temperature then, you want to absorb that heat, according to Le Chatelier's principle, and so that would favor a shift toward products.
So let's look some more at this. The predictive tool here is delta h. So delta h, whether the reaction is exothermic or endothermic, is going to be a predictive tool in thinking about the direction of change, when heat is added to a system or heat is removed from a system.
So let's try this out. Heat is added to a system. You're given information about your predictive tool, delta h. Which direction will the reaction go? All right, 10 seconds. Why don't you discuss with your friends and vote again. All right, 10 more seconds. Yes! I knew we could get into the 90's. All right, so what type of reaction is this, endo or exothermic? Exothermic. And so we're adding heat to an exothermic reaction, so it wants to shift to absorb that heat, so it wants to go in endothermic direction, which is the reverse direction or toward the reactants. And that's how it's supposed to work too, more people get the right answer after the group discusses.
All right. So we've been talking about the equilibrium constant so far in terms of it being a constant, it's called the equilibrium constant, but it's only constant at a given temperature. So the equilibrium constant changes with temperature. It's also true that rates of reaction change with temperature. And kinetics is our last unit in this course, and we'll be discussing that quite a bit. So how does the equilibrium constant change with temperature? Let's consider that.
So let's look at some of the equations that we know that have delta h in them -- delta h, again, is our predictive factor when we're talking about changes in temperature. We know that delta g nought is minus r t natural log of k, so the relationship with our standard free energy, our gas constant temperature, and our equilibrium constant. We also know that delta g nought equals delta h nought minus t delta s nought. If those equations don't look familiar, then you should go back and review the material on thermodynamics because you're going to need it in this unit, the next unit, the unit after that, and then the next two units, and they we're done with the class. All right, so good to get familiar with this now.
All right, so we can rearrange these in terms of solving for our equilibrium constant. And so we see the natural log of the equilibrium constant minus delta h nought over r t, plus delta s nought over r. And so, it's reasonable to assume that for the things we're talking about, that delta h nought and delta s nought are pretty much independent of temperatures. That would be true for pretty much any temperature we would be talking about. So that means that k, or the natural log of k, is going to change depending on the temperature. It won't change -- these other ones are constant at all the temperatures, so there's a relationship between equilibrium constant and temperature.
So you can consider an equilibrium constant at one temperature, and an equilibrium constant at another temperature. So we can talk about the natural log of a k 2, of an equilibrium constant 2, equal to minus delta h over r t temperature 2, plus delta s nought over r. We can do the same thing for another temperature, another temperature 1, equilibrium 1, and if we subtract these two, delta s is going to cancel out, and we're going to get this equation, which you know is important because it has a name, so this is the van't Hoff equation. And at some point later in the course, I'll be asking you to come up with this name, so I always get very excited when people can tell me it's a van't Hoff equation later on, not that that's on a test, but there are very few equations that have names.
So here we go. So what does this do? Well, we can talk about the natural log of one equilibrium constant over another, an equilibrium constant k 2, which is the equilibrium at temperature 2, equilibrium constant k 1, which is the equilibrium constant at temperature 1, is going to be equal to minus delta h nought over r, and then it depends on the temperature. So you bracket 1 over temperature 2 minus 1 over temperature 1. And this just comes from subtracting those 2 equations. So again, you can think about how the equilibrium constant's going to change with temperature -- that's what this equation does for you.
So let's look at some of the things that fall out of this particular equation. Let's think first about a case where this delta h nought is less than zero. What type of reaction is that? Exothermic. All right, so now let's consider a case for this exothermic reaction where we're going to increase the temperature, and so that means that t 2 will be greater than t 1 -- we've increased the temperature. So now let's think about what sign the natural log of k 2 over k 1 will have if these things are true. So there's a minus in the equation, let's put the minus down here. Delta h nought is negative, it's exothermic reaction, so that's another negative sign. And if we're increasing the temperature, and t 2 is greater than t 1, then this temperature term will also have and negative sign. So if you have negative times negative times negative, you're going to get a negative for your net result over here. And what that will mean in terms of k 2 and k 1, is that k 1 will be greater than k 2.
So you can think about this mathematically by running through this argument. You can also sort of think about it in terms of what you would expect if you increase the temperature in terms of what's the relative ratio of products to reactants at equilibrium for an exothermic reaction. So if you're increasing the temperature of an exothermic reaction, the reaction would want to shift in the direction to absorb that heat in the endothermic direction. So you would expect that the equilibrium constant that was at the lower temperature would be larger than the higher temperature one, that there'd be less products at this new higher temperature.
So again, you can think about it in terms of Le Chatelier's principle, or you can run through with this equation and do the math and think about the relative size of k 1 and k 2.
So now let's think about what happens when you decrease the temperature where t 2 is less than t 1. So we can do the same thing, we have a negative sign here, we also have a negative sign for delta h, it's an exothermic reaction. But now we have a positive sign for this temperature term. So overall we're going to have a positive sign for the natural log of k 2 over k 1, which mathematically is going to mean that k 1 is less than k 2 or that you would expect more products in the equilibrium constant at higher temperature.
All right, so that's an exothermic reaction. So if you're ever having problems with Le Chatelier, you can go and use the van't Hoff equation to think about what you would expect in terms of this shift, what you would expect if you change the temperature of an exothermic reaction in terms of the magnitude of the new equilibrium constants.
All right, now you can do the same for me in terms of this delta h nought is greater than zero. Which of the following are going to be true, and notice there's a possibility that all of them are true or that just some of them are true or that one of them is true. All right, take 10 more seconds. See, actually people did very well, because e is also true, all of those are true, but d was also true. So if we add the 30 and the 58 together, I'm very happy.
All right, so let's look at this -- we'll keep the questions up and let's consider all of them in order. So the first one is that the reaction was endothermic, I think everybody saw that as being correct. OK, the second one talked about the equilibrium constant is higher at higher temperatures. So let's look at the first one. We increased the temperature here, and we see that if you work out the math or just think about it, k 2 is larger than k 1, so the second equilibrium constant is greater than the first when you increase the temperature. So that would favor then, in an endothermic direction, if you're increasing the temperature, it favors the endothermic direction of the reaction, so you could think about the ratio having more products at that new equilibrium constant at that higher temperature. So that one was also true.
Then let's think about when you decrease the temperature, when temperature 1 is greater than temperature 2. And so this would favor, then, the exothermic direction. So you would expect less products at this newer temperature. So, this part asked about k 1 versus k 2, and we see k 1 is greater than k 2. And then d says there's fewer products and equilibrium when the temperature is decreased, and that's just the word way of saying the same thing. So all of those are true. But again, people did very, very well with this question.
All right. So now we're going to combine what we learned on Friday with what we learned today, and think about ways that that can be applied. So why should you care about Le Chatelier's principle? Well, for two reasons -- one I think Le Chatelier had sort of a good life plan that when stress is applied to the system, one should respond in a way to minimize that stress. I think that is an important life lesson. But also, it's very useful in terms of thinking about maximizing a yield of a reaction. So if you were going to create an industrial process and make lots of money and give some of it back to MIT to improve things in terms of teaching chemistry at MIT, then you would want to think about these principles, because you would want to maximize your yield. If you're going to be making a product, you want to make a lot of that product, and so you want to be thinking about these things.
So we've talked about the reaction with nitrogen and hydrogen making ammonia last time. And this is an exothermic reaction. So, there are a lot of people who want to make ammonia -- it's for fertilizer, and as some of you have heard in terms of terrorism, it also can be used as an explosive, so there are a lot of people who want to make a lot of this. So how do you maximize this yield? It's an exothermic reaction. So you can think about temperature.
So here, low temperature would favor product, which is good. We haven't talked about kinetics yet, but low temperature also slows the rate, which is bad. Because you not only want to make a lot of your product, you want to make it in a reasonable time frame and sell it and make lots of money and retire early. So you care about how fast the reaction is going as well.
So for an exothermic reaction then, you have to balance out what's thermodynamically favorable and what's kinetically favorable. So thermodynamically, you would want a low temperature, but kinetically in terms of the rate, that's not so good. So they use a compromised temperature, which is 500 degrees c, so that's pretty high actually for this reaction. So what are other things that you could do to drive this reaction toward products? So yeah, what's something else you could do?
STUDENT: Increase the volume.
PROFESSOR: Right. So you could think about volume here. So here we have four molecules of gas on one side, and two molecules of gas on the other side. So you can think about changing that to favor your products. What is something else you could do?
STUDENT: Enzymes.
PROFESSOR: Enzymes, we'll talk about that in a minute. If you can't use the enzymes, what's something else that you could do?
STUDENT: You could remove ammonia from the system.
PROFESSOR: Yup, so you could remove ammonia from the system, which would also shift the direction. So let's just put those two things down. Remove products. So if you remove ammonia from the system, and they actually do this in the industrial process, so they'll stop -- they'll, at a certain time points, they will remove the product and they'll say hey, let's shift the reaction to make more. You just changed q versus k, you removed your product, so now we want to shift to minimize that stress and make more product. And the first response, compress the volume, so we go from four molecules to two molecules, and that would be favor -- again, the system would want to respond, you compressed the volume, you increased the pressure, so you want to respond in such a way that compensates for that increased pressure, which is decrease the pressure by going from the four molecules to two. So both of those are great, and both of those are, in fact, used.
Sometimes when you study chemistry you'll realize that some of the -- people made a lot of money on this, and that some of the principles are actually very simple. You are learning principles in this class that, applied correctly, could make you a lot of money. Some of the scientific discoveries are actually not all that complicated.
All right. So someone said use enzymes. I love that answer, I want to use enzymes to do a lot of those things, and people are trying to do this. Now, why would you want to use an enzyme? Well, there is a lot of nitrogen in the air, but there's not a lot of usable nitrogen, because n 2 gas is in the air, but it's very difficult to break it apart to form ammonia. And when you think about bonding, you can probably tell me why it's hard to break n 2 apart, and if you consider how many bonds would be between those two nitrogens. So it's there but it's hard to do.
So the industrial process that's used now pretty much is the same industrial process that's been used for quite a while, the Haber-Bosch process, which does not use enzymes. And here are some pictures of these folks. This resulted in two Noble prizes, the development of the industrial process here, and it's still being used. On a historic note, these were German scientists, and so, working out this process was important to the Germans during World War II. It's still the process going on today.
So what about enzymes. Maybe enzymes could do a bit better than this process. It's not true yet. Still the Haber-Bosch industrial process is the one that's being used, but enzymes can do it. So, some of the problems of a lot of the common industrial reactions are that you use high temperatures -- remember we said the compromised temperature was 500 degrees celsius. We were talking about compressing the volume, so you want to put in energy to compress that volume. And so that is expensive. And you might want to think about a way, could you do that reaction at normal ambient temperature without applying energy to the system.
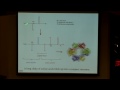
Well, enzymes can do this reaction at ambient temperatures and they don't have to have high pressure associated with them, and the way the enzymes do this, and here's a little cartoon of a space-filling model of a particular enzyme called nitrogenase. So for nitrogen, and the ase means it's an enzyme. And the secret to this enzyme, how it can do what Haber and Bosch got two Nobel prizes for -- this enzyme has received no Nobel prizes, and it can do the same thing. Its secret are metals. It uses these clusters of metals in the context of the protein environment to do this chemistry, and they have iron, and they have also inorganic sulfide, and molybdenum, and so these combinations of metals can do this chemistry. And a number of scientists have been working for decades now trying to understand how the enzyme works. It's actually quite complicated and it's been pretty controversial.
So let me just kind of show you at the heart of the enzyme, here all the atoms of the enzyme, but if you rotate the enzyme around and go in, the secret was that those combinations of metals. And one of the units that we're going to have second to last in this courses is about transition metals, that metals can do a lot of really important things in biological systems, and also in industrial processes and other things as well.
So I do also like to mention research is going on here at MIT. I've mentioned Dick Schrock before, he won a Nobel Prize in chemistry a few years back, but not for his work on sort of nitrogenase systems, but he was able to design a small catalyst that can do this chemistry, that can catalyze this reduction of nitrogen to ammonia at a defined molybdenum center. Again, we'll talk about transition metals later in the course. And so his laboratory is very interested in coming up with better ways to split nitrogen than the industrial processes that are currently used. Some people are studying enzymes, other people are trying to use some of the tools that come out the enzymes, thinking about the enzymes and come up with other catalysts as well. So this work is going on here at MIT.
All right, so let's give one more example of Le Chatelier from a biological perspective, and this has to do with the following reaction. The combination of oxygen with hemoglobin in your blood. And the reaction here, we have hemoglobin plus oxygen has oxyhemoglobin, so the oxygen is now bound to be hemoglobin. This reaction is very important for you. None of us would be alive if this was not happening. We need to get the oxygen from our lungs to our cells.
So what happens if you decide to climb Mount Everest, for example. You may be thinking how hard can that be after a first semester freshman year at MIT. Really, I can think more broadly about it. All right, how many of you have climbed a mountain so far? Woah, a lot of people. OK, something about going to MIT and climbing big mountains seems to be connected. How many people feel like after surviving the first year, why not, go ahead and climb a mountain? Any more people? OK, no. So you gotta -- you've been through the hard part now, you don't want to risk anything anymore.
All right, so this slide seems to be cutting off some stuff, so let's look over here. So what happens to this reaction? Well, as you go up higher and higher, the partial pressure of oxygen changes. So you're going to drop in partial pressure 0.2 to 0.14 . So what happens to this reaction if this value decreases? What happens, what direction does it shift? For products or reactants? Right. So, if this is going down, then some of your oxygenated hemoglobin is going to a release its oxygen. So it'll respond in such a way to compensate for that added stress.
So what can you do to switch it back the other way? Again, we want our blood to be oxygenated. What happens to compensate for this if you're going to climb a mountain? What happens in the body? Yeah.
STUDENT: The body can produce more hemoglobin.
PROFESSOR: The body can produce more hemoglobin. So you often go to another altitude and hang out for a while before you start going way up. And what's happening during that time is your body realizes there is a problem, it knows about the Le Chatelier's principle, and so it's going to make more hemoglobin. And if you add more hemoglobin to this reaction, what direction does it go? Toward products, right.
OK. So I wanted to mention significant figures, and there is -- I have to make a confession that when I first started teaching this course, I had never really paid attention to the rules of significant figures for logs. In this unit, the next unit, the unit after, you get the idea, there are going to be a lot of logs, and they have special rules for significant figures. So in the bottom of your handout, these rules are explained, it's also in your book. Pay attention to this. It's going to help you on all the problem-sets and in the course. So I just wanted to point out special rules about significant figures for logs.
All right, that's it for today, see you on Wednesday.
Free Downloads
Free Streaming
Video
- iTunes U (MP4 - 110MB)
- Internet Archive (MP4 - 110MB)
Caption
- English-US (SRT)